Cite this Article
Niu Xiaoqing, Xie Min, Zhou Fen, Mu Rende, Song Xiwen, An Shengli. Substituent Influence of Yttria by Gadolinia on the Tetragonal Phase Stability for Y2O3–Ta2O5–ZrO2 Ceramics at 1300 °C . Journal of Materials Science & Technology, 2014, 30(4): 381-386  
Permissions
Copyright©2014, Editorial Board of Journal of Materials Science & Technology
Copyright 2014, Editorial Office of Journal of Materials Science & Technology
Substituent Influence of Yttria by Gadolinia on the Tetragonal Phase Stability for Y2O3–Ta2O5–ZrO2 Ceramics at 1300 °C
Niu Xiaoqing1, Xie Min1, Zhou Fen1, Mu Rende2, Song Xiwen1,*, An Shengli1
1. Inner Mongolia Key Laboratory of Advanced Ceramics and Device, School of Materials and Metallurgy, Inner Mongolia University of Science and Technology, Baotou 014010, China
2. Beijing Institute of Aeronautical Materials, Beijing 100095, China
Corresponding author. Prof., Ph.D.; Tel.: +86 472 6896122; Fax: +86 472 5951571.
Abstract

The influences of equimolar substitution of yttria by gadolinia on the phase constituent and stability for Y2O3–Ta2O5–ZrO2 ceramics have been investigated. The ceramics with the Gd content lower than 8 mol% exhibit pure tetragonal phase as well as high tetragonal phase stability. However, the high Gd containing ceramics consist of t-ZrO2, m-ZrO2 and monoclinic GdTaO4 and show complicated phase evolution behaviors. The fractions of monoclinic ZrO2 and GdTaO4 increase with increasing Gd content, indicating that the excessive substitution of Gd for Y results in a reduction in the tetragonal phase stability. In addition, the lower Gd containing ceramics show an almost invariable tetragonality, while the higher Gd containing ceramics reveal a composition-dependent tetragonality. Accordingly, an association of the tetragonal phase stability and tetragonality with the Gd concentration is established, which provides us a clue to understand the phase stability of these ceramics.

Keyword: Ceramics; ZrO2; X-ray diffraction; Thermal stability
1. Introduction

Partially stabilized zirconia with tetragonal structure is of great significance for high temperature applications due to its high strength, excellent fracture toughness, low thermal conductivity, relatively high thermal expansion coefficient and good erosion resistance [1]. 6–8 wt% Y2O3 partially stabilized zirconia (6–8YSZ) with the tetragonal structure is widely used as a topcoat ceramic of thermal barrier coatings. However, the tetragonal phase is unstable during long-term exposure above 1200 °C. The high temperature exposure leads to the conversion of the tetragonal phase to a low-yttria tetragonal phase and a high-yttria cubic phase. The low-yttria tetragonal phase will further transform to a monoclinic phase on cooling [2] and [3]. Accordingly, it is unsuitable for the applications at temperatures higher than 1200 °C.

Unlike YSZ, the tetragonal phase of ZrO2 stabilized with equimolar of YO1.5 and TaO2.5, as the content of the stabilizers YO1.5 and TaO2.5 is in the range of 16–22 mol%, exhibits high phase stability at 1500 °C. It does not transform to m-ZrO2, even in a wide range of grain size or aging temperature [4] and [5]. Furthermore, dissimilar to YSZ, whose tetragonal structure is only stabilized by oxygen vacancies [6], ZrO2–Ta2O5–Y2O3 compositions contain almost no oxygen vacancies, suggesting a difference in the stabilization mechanism. It has been found that the transformability of tetragonal phase into monoclinic phase for YSZ ceramics is dependent on its tetragonality [7] and [8]. The tetragonal phase stability is improved by decreasing tetragonality [8] and [9] and the largest possible tetragonality of the nontransformable tetragonal phase is 1.0203 [4]. However, ZrO2–Ta2O5–Y2O3 compositions exhibit an extraordinarily high tetragonality, commonly higher than 1.0250 [10] and [11]. The extraordinarily high tetragonality is considered to be associated with the high stability of the tetragonal phase. Unfortunately, the relationship between the phase stability and the high tetragonality for these compositions has been less well understood.

It is well known that the tetragonal phase stability of YSZ can be improved by utilizing alternative stabilizers [12] and [13]. Rebollo et al. [12] and [14] investigated the effect of Ln2O3 ( Ln = Yb, Gd, Sm, Nd) on the phase stability of the tetragonal phase, herein the series with 3.8 mol% Y2O3 + 3.8 mol% Ln2O3 have better resistance to destabilization than YSZ and the stability is independent of the cation radius. Jones et al. [13] and [15] found that Sc2O3–Y2O3–ZrO2 has significantly higher tetragonal phase stability than Y2O3–ZrO2. Gd2O3 is in general a less effective stabilizer than Y2O3, whereas the partial substitution of Gd2O3 for Y2O3 does enhance the phase stability when Y2O3 remains the dominant stabilizer [16] and [12]. With increasing Gd2O3 content, the phase constitutions of Y2O3–Gd2O3–ZrO2 will gradually evolve from tetragonal to the mixture of tetragonal and cubic, finally to the cubic [16] and [17]. For the Y2O3–Ta2O5–ZrO2 system, however, the association of the tetragonal phase stability with the substitution of Y2O3 by other rare earth oxides has not been reported.

Based on the above mentioned issues, the main objective of the present work is to evaluate the substituent influence of Gd2O3 for Y2O3 on the tetragonal phase stability of the Y2O3–Ta2O5–ZrO2 system. To achieve the goal, the equimolar substitution of Y2O3 with Gd2O3 was selected to change the average size of cations and thereby to alter the tetragonality of these ceramics. In addition, it has been found that a single stable tetragonal phase of TaYSZ presents only at and above 1500 °C and the decomposition reaction occurs below 1500 °C [18] and [19]. Thus, the appraisement of the tetragonal phase stability at temperatures below 1500 °C is worthful. Accordingly, an annealing temperature of 1300 °C was chosen.

2. Experimental
2.1. Material preparation

The powders for GdO1.5–YO1.5–TaO2.5–ZrO2 samples were synthesized by solid-state reaction of well mixed stable oxides. The starting materials used in this study were rare earth oxides Gd2O3 (99.95%), Ta2O5 (99.9%), Y2O3 (99.95%) and ZrO2 (99.9%). All powders of these oxides were fired at 800 °C for 3 h before weighting. The mixed oxides with the corresponding amount were ball-milled by high-purity YSZ balls and polyurethane containers for 24 h in a planetary mill. The achieved suspensions were dried completely, and then calcined at 1500 °C for 6 h for the solid-state reaction. The calcined powders were ball-milled in ethanol for 24 h again and dried at 70 °C for 24 h to evaporate ethanol. The resultant powders were uniaxially die-pressed under 20 MPa, followed by cold isostatic pressing under 200 MPa into pellets. The green pellets were sintered at 1600 °C for 5 h in air.

2.2. Material characterization

The phases of the samples were characterized by powder X-ray diffraction (XRD, Bruker, D8 Advance) with Cu Kα radiation. To determine the lattice parameters, XRD patterns were recorded by a slow scanning over the range 20°–80° with the rate of 0.02°/s. Raman spectra were performed at the excitation with an argon ion laser of 488 nm (Coherent, Santa Clara, CA) and collected with a microscope-based Raman spectroscopy system (Triple monochromator Jobin–Yvon, Edison, NJ.) at a rate of 600 cm-1/30 s. The wavenumber values of the Raman spectra are accurate to 1 cm-1. The tetragonal phase stability of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics was investigated by heating the samples in a high-purity Al2O3 crucible to 1300 °C, and holding for 50 and 100 h with the heating and cooling rates of 10 °C min-1. X-ray analysis of the annealed samples was performed by using the same method described above. The strain of the tetragonal phase for the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics was estimated from Hall's equation [20] using the t(002), t(200), t(004) and t(400) reflections.

(βhklcosθ)/λ=1/Dhkl0+ηhkl(sinθ/λ) (1)

where Dhkl0 is the effective crystallite size in the < hkl>direction and η hkl is the strain in that direction and its value is acquired by linearly fitting the relationship of ( β hklcosθ) vs (sinθ/ λ).

The bending strength of these ceramics was tested by the three point bending method using an electric universal testing machine (CSS-88010, Chanch Testing Equipment Research Institute). The dimension of the samples is 40 mm × 6 mm × 6 mm and the loading speed is 0.5 mm min-1.

3. Results and Discussion
3.1. Structure of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics

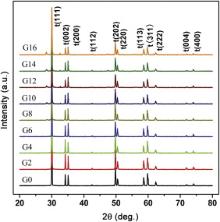
The XRD patterns of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics are shown in Fig. 1. In the {400} region of 2 θ = 70°–76°, all the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics only display double split peaks, t(004) and t(400), which is the characteristic of the tetragonal phase. This suggests that all these series do not contain cubic phase. In the {111} region (2 θ = 27°–34°), the ceramics with lower gadolinia concentration (0, 2, 4, 6 mol%) exhibit no detectable peaks of the monoclinic phase, whereas those with higher gadolinia concentration (8, 10, 12, 14, 16 mol%) exhibit two extra peaks at around 28.5° and 33°, indicating the existence of the monoclinic phase. Combined with the appearance of the extra peak at around 48°, the monoclinic phase can be assigned to YTaO4 or GdTaO4. This result clearly suggests that the monoclinic GdTaO4 will precipitate from the tetragonal zirconia for these ceramics with the gadolinia content higher than 8 mol%.

Fig. 1. XRD patterns of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics.

The calculated lattice parameters of the tetragonal and monoclinic phases for the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics are listed in . The lattice parameters a, c and cell volume of the tetragonal phase do not show regular variations with the composition. However, the tetragonality c/ a (cell parameters ratio) is strongly composition-dependent. The tetragonality is almost constant for the ceramics with the Gd content lower than 8 mol%, whereas it gradually decreases with increasing Gd content for those with the Gd content higher than 8 mol%. This is quite dissimilar to the binary systems of zirconia doped by trivalent rare earth and alkaline-earth element oxides. In the binary systems, the tetragonality is independent of the dopant species, but dependent on the dopant content [7] and [8]. When the dopant content is increased, the tetragonality of t-ZrO2 decreases toward unity, which corresponds to c-ZrO2. The tetragonality of t-ZrO2 in the binary systems represents a degree of cubic fluorite structure distortion or microstrain due to the lattice anisotropy. Accordingly, the reduction in the tetragonality by an increased amount of stabilizer means an enhancement in the phase stability of t-ZrO2 [3] and [21]. For the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics with higher Gd content, the composition-dependent tetragonality should be associated with the reduction in the content of the trivalent and pentavalent oxides dissolving in the tetragonal zirconia phase due to the improvement in the mass fraction of the monoclinic GdTaO4. This suggests that the variation in the tetragonality with the dopant content for these ceramics is opposite to that for the binary systems of ZrO2. Hence, the extraordinarily high tetragonality of these ceramics is not in relation to a distorted form of the cubic structure. In addition, the reduction in the tetragonality of the tetragonal phase with the composition implies a fine variation in the crystal structure.

Table 1. Compositions and physical properties for GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics

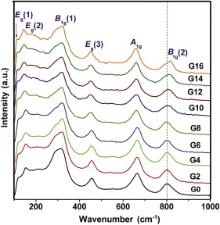
The Raman spectroscopy was performed to give a further insight into the structure of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics, as depicted in Fig. 2. These ceramics only contain six Raman active vibration modes, which are characteristic peaks of the tetragonal phase. This indicates that the monoclinic GdTaO4 does not display new Raman active vibration mode, which is in accordance with the result reported by Shen [10]. The primitive cell of the tetragonal zirconia contains two formula units. Oxygen atoms are located in 4 d ( C2 v) special position and zirconium atoms are in 2 a ( D2 d) special position. Group theoretical analysis gives the following normal Raman active vibration modes [22]:

Γ( R)= A1g+2 B1g+3 Eg (2)

where A1g, B1g(1) and B1g(2) are correlated with three stretching vibration modes, and Eg(1), Eg(2), Eg(3) are associated with three bending vibration modes. The B1g(2) mode shifts towards a higher frequency (blue shift) for the series with the Gd content higher than 8 mol%, demonstrating an enhancement in the Zr–O stretching mode and thereby a shortening in the bond length. The other modes exhibit a composition-independent Raman frequency. These results are in accordance with those predicted by the XRD analysis. In addition, the regular shift of B1g(2) mode is usually associated with the changes of lattice strains, mode softening or inter-atomic spacing induced by pressure or an external stress [23]. In this work, it is improbable that the shift originates from external imposed strains. Most likely, the shift is a result of internal mismatch strains generated by the phase separation into a mixture of monoclinic and tetragonal phases.

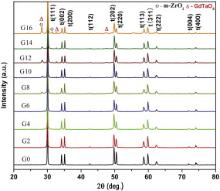
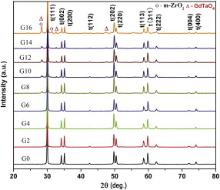
Fig. 2. Raman spectra of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics.

3.2. High temperature phase stability of GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics

To evaluate the stability of the tetragonal phase, GdO1.5–YO1.5–TaO2.5–ZrO2 samples were annealed at 1300 °C for different duration. The XRD patterns of the samples annealed for 50 and 100 h are shown in Fig. 3 and Fig. 4, respectively. It clearly demonstrates that the phase evolution is greatly associated with the composition and the annealing time. The ceramics with lower Gd content (0, 2, 4, 6 mol%) still consist of the pure tetragonal phase, and no any extra phase appears even after annealing for 100 h. This indicates that the lower Gd containing ceramics have high tetragonal phase stability. However, the ceramics with higher Gd content (8, 10, 12, 14, 16 mol%) exhibit a complicated composition-dependent decomposition behavior. After annealing at 1300 °C for 50 h, the amount of the precipitated monoclinic GdTaO4 increases. Moreover, m-ZrO2 occurs in the ceramics with the Gd content higher than 12 mol%. After annealing at 1300 °C for 100 h, the m-ZrO2 has been found in all the higher Gd containing ceramics. These results suggest that Gd2O3 has a significant and complicated effect on the stability of the tetragonal phase for these ceramics. When the molar fraction of Gd2O3 substituting for Y2O3 is lower than 0.5, Gd2O3 has at least no disadvantageous effect on the stability of the tetragonal phase. However, as the substituting molar fraction is above 0.5, the resistance to destabilization of the tetragonal phase gradually reduces with increasing Gd content.

Fig. 3. XRD patterns of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics annealed at 1300 °C for 50 h.

Fig. 4. XRD patterns of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics annealed at 1300 °C for 100 h.

In addition, the mass fraction of the monoclinic GdTaO4 precipitation improves with increasing Gd content, indicating that the content of the stabilizers Gd and Ta in the tetragonal phase decreases with increasing Gd content. This demonstrates that the solubility of GdTaO4 in the ZrO2 matrix is lower than 16 mol%. When the sample is isothermally annealed at 1300 °C, the mass fraction of GdTaO4 substantially increases with prolonging heat treatment duration, concurrently, m-ZrO2 is precipitated in all the higher Gd containing ceramics and its mass fraction increases with the Gd content. These results indicate that Gd2O3 does promote the destabilization of the tetragonal phase when its content exceeds that of Y2O3.

The phase evolution behavior of GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics is clearly dissimilar to that of YSZ. It has been suggested that the tetragonal phase of YSZ undergoes a diffusion-controlled partitioning to the Y2O3-poor tetragonal and Y2O3-rich cubic phases at temperatures higher than 1200 °C, followed by the tetragonal phase undergoing the deleterious transformation to the monoclinic phase on cooling [24] and [25]. For these GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics, however, the partitioning of the tetragonal phase contains a process of the monoclinic GdTaO4 precipitation and a subsequent process of the transformation from tetragonal to monoclinic phase. The addition of a large mismatch cation, such as Gd3+, can provide a high driving force for the partitioning of the nontransformable tetragonal phase [26], resulting in a decrease in the resistance to destabilization of the tetragonal zirconia. This phase evolution is distinct from a nucleation and growth process. In phase evolution by nucleation and growth, the compositions of the precipitating phases and the matrix do not have significant changes. Hence, it can be suggested that the phase separation within the tetragonal region of the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics is consistent with the coherent phase separation, which is similar to the tetragonal-cubic phase separation in yttria-stabilized zirconia in the form of spinodal decomposition [27] and [28].

The above results provide a clue for understanding the phase stability of GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics. Furthermore, the strain energy would dominantly contribute to the stabilization of tetragonal phase [29], thus the variation of strains with the Gd content is evaluated and the result is shown in Fig. 5. The uncertainties from the strain calculation according to Eq. were estimated to be less than 6%. As the results shown, the strain increases with increasing Gd2O3 content. The increase in the strain should be attributed to the lattice distortion promoted by the Gd3+ cation. This corresponds to the explanation of the composition-dependent stability of the tetragonal phase. The transformation from tetragonal to monoclinic phase is thermodynamically governed by the following relation [30]:

ΔGt-m=ΔGC+ΔUSE+ΔUS (3)

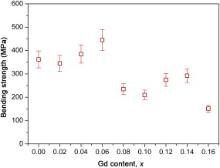
where Δ GC(<0 at temperatures below the equilibrium MS temperature) is the difference in chemical free energy between the tetragonal and monoclinic phases. Δ USE refers to the change in elastic strain energy associated with the transformation. Δ US is the change in energy associated with the formation of new interfaces when the transformation occurs. It remains in its tetragonal state if the overall thermodynamic driving force Δ Gt-m>0. When Δ Gt-m becomes negative, the tetragonal phase is metastable or unstable and may transform into its monoclinic structure. The elastic self-energy Δ USE is directly influenced by applied or internal stresses: tensile stress will act to reduce Δ USE, destabilizing the tetragonal phase, whereas hydrostatic pressure favors the retention of the tetragonal phase. Accordingly, the enhancement in the strain energy should dominantly contribute to the reduction in the phase stability for the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics. In addition, this leads to a corresponding variation in the mechanical property, as shown in Fig. 6. As the Gd2O3 content increases from 0 to 6 mol%, the bending strength of the ceramics increases firstly. With a further increase in Gd2O3 content, the bending strength decreases. This is in accordance with the results of the phase stability found in the GdO1.5–YO1.5–TaO2.5–ZrO2ceramics.

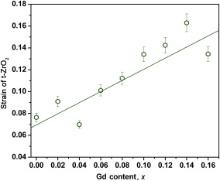Fig. 5. Variation of the strain with the gadolinium concentration for the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics.

Fig. 6. Variation of the bending strength with the gadolinium concentration for the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics.

3.3. Relationship between tetragonality and tetragonal phase stability

In order to give an insight into the intriguing composition-dependent features for GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics, the variations of the tetragonality with the gadolinium concentration was reevaluated, which is shown in Fig. 7. It is obvious that the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics exhibit a composition-dependent tetragonality and there exists an upper limit of the substitution amount of Gd2O3 for Y2O3, which is in the range of 6–8 mol%. When the substitution amount is below this upper limit, the tetragonality is almost composition-independent, suggesting the tiny variation in the structure for these ceramics. In this region, the ceramics display high tetragonal phase stability, thus denote it as tetragonal phase stability region. However, when it is above the limit, the tetragonality regularly decreases with increasing gadolinium concentration, which means a sequential composition-dependent variation in the structure. In this region, the tetragonal phase is inclined to decompose, denoting it as tetragonal phase decomposition region. Taking into account the fact that the monoclinic phases precipitate only as the substitution amount is higher than 8 mol%, it can be concluded that there exists a lower limit of the tetragonality. The compositions with tetragonality higher than the limit have high tetragonal phase stability, and those with the tetragonality lower than the limit are inclined to decompose. The decrease in the stability of the tetragonal phase for higher Gd containing ceramics is associated with the reduction in the tetragonality. Furthermore, the increment in the mass fraction of the precipitated monoclinic phases with the gadolinium content means a decrease in the molar fraction of the stabilizers in the tetragonal phase. Accordingly, the reduction in the tetragonality is caused by the decrease in the content of the stabilizers. That is, the tetragonality for these GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics will be improved as the stabilizers content increases. This aspect is quite different from the binary systems of zirconia doped by trivalent rare earth and alkaline-earth element oxides. In the binary systems, the tetragonal phase whose c/ a larger than 1.0203 is transformable and that whose c/a smaller than 1.0203 is nontransformable. The resistance to the decomposition of the tetragonal phase enhances with decreasing tetragonality, as a result of increasing stabilizer content [31]. The particular features of these compositions provide us a promising approach to optimizing these equimolar trivalent and tentavalent oxides codoped tetragonal zirconia systems. However, further detailed experiments and theoretical proofs should be given for this.

Fig. 7. Variation of the tetragonality with the gadolinium concentration for the GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics.

4. Conclusion

For these GdO1.5–YO1.5–TaO2.5–ZrO2 ceramics, the phase structure as well as phase stability are consistently associated with the composition. The lower Gd containing ceramics display an almost composition-independent tetragonality, high resistance to the tetragonal phase decomposition. For the ceramics with higher Gd content, however, the tetragonality of t-ZrO2 gradually reduces with increasing Gd content, which is as a result of the reduction of the Gd content in the tetragonal phase. Moreover, these high Gd containing ceramics show a great composition-dependent phase evolution behavior. A lower limit of the tetragonality is introduced to judge the tetragonal phase stability. The ceramics whose tetragonality is higher than the lower limit is of the tetragonal phase stability, however those with the tetragonality lower than the lower limit are subject to decompose. An association of the phase structure, stability and the composition is established, which is beneficial to optimizing these ceramics.

Acknowledgments

The authors gratefully acknowledge the financial support for this research by the National Natural Science Foundation of China under Grant No. 50974074, the Program for New Century Excellent Talents in University under Grant No.NCET-10-0910 and the Natural Science Foundation of Inner Mongolia under Grant No. 2011ZD09.

The authors have declared that no competing interests exist.

Reference
1. A. G. Evans, A. H. Heuer, J. Am. Ceram. Soc. , 63 (1980), pp. 241–248 [Cited within: 1] [JCR: 2.107]
2. U. Schulz, J. Am. Ceram. Soc. , 83 (2000), pp. 904–910 [Cited within: 1] [JCR: 2.107]
3. R. A. Miller, J. L. Smialek, R. G. Garlick, First International Conference on the Science and Technology of Zirconia, Science and Technology of Zirconia I, American Ceramic Society, Cleveland , OH (1980), pp. 241–253 [Cited within: 2]
4. D. J. Kim, J. Am. Ceram. Soc. , 73 (1990), pp. 115–120 [Cited within: 2] [JCR: 2.107]
5. D. J. Kim, T. Y. Tien, J. Am. Ceram. Soc. , 74 (1991), pp. 3061–3065 [Cited within: 1] [JCR: 2.107]
6. S. Fabris, A. T. Paxton, M. W. Finnis, Acta Mater. , 50 (2002), pp. 5171–5178 [Cited within: 1] [JCR: 3.941]
7. M. Yashirna, T. Noma, N. Ishizawa, M. Yoshimura, J. Am. Ceram. Soc. , 74 (1991), pp. 3011–3016 [Cited within: 2] [JCR: 2.107]
8. K. A. Khor, J. Yang, Scr. Mater. , 37 (1997), pp. 1279–1286 [Cited within: 3] [JCR: 2.821]
9. K. A. Khor, J. Yang, Mater. Lett. , 31 (1997), pp. 23–27 [Cited within: 1] [JCR: 2.224]
10. Y. Shen, R. M. Leckie, C. G. Levi, D. R. Clarke, Acta Mater. , 58 (2010), pp. 4424–4431 [Cited within: 2] [JCR: 3.941]
11. X. Song, M. Xie, R. Mu, F. Zhou, G. Jia, S. An, Acta Mater. , 59 (2011), pp. 3895–3902 [Cited within: 1] [JCR: 3.941]
12. N. R. Rebollo, O. Fabrichnaya, C. G. Levi, Z. Metallkd, 94 (2003), pp. 163–170 [Cited within: 3] [JCR: 0.857]
13. R. L. Jones, D. Mess, Surf. Coat. Technol. , 86–87 (1996), pp. 94–101 [Cited within: 2] [JCR: 1.941]
14. N. R. Rebollo, A. S. Gand hi, C. G. Levi, Proceedings of the Electrochemical Society: High Temperature Corrosion and Materials Chemistry IV, Electrochemical Society (2004), p. 431 [Cited within: 1]
15. R. L. Jones, R. F. Reidy, D. Mess, Surf. Coat. Technol. , 82 (1996), pp. 70–76 [Cited within: 1] [JCR: 1.941]
16. A. Portinha, V. Teixeira, J. Carneiro, M. F. Costa, N. P. Barradas, A. D. Sequeira, Surf. Coat. Technol. 188–189 (2004), pp. 107–115 [Cited within: 2]
17. Z. G. Liu, J. H. Ouyang, Y. Zhou, Mater. Lett. , 62 (2008), pp. 3524–3526 [Cited within: 1] [JCR: 2.224]
18. F. M. Pitek, C. G. Levi, Surf. Coat. Technol. , 201 (2007), pp. 6044–6050 [Cited within: 1] [JCR: 1.941]
19. A. Bhattachaya, V. Shklover, K. Kunze, W. Steurer, J. Eur. Ceram. Soc. , 31 (2011), pp. 2897–2901 [Cited within: 1] [JCR: 2.36]
20. T. Mitsuhashi, M. Ichihara, U. Tatsuke, J. Am. Ceram. Soc. , 57 (1974), pp. 97–101 [Cited within: 1] [JCR: 2.107]
21. J. R. Brand on, R. Taylor, Surf. Coat. Technol. , 46 (1991), pp. 75–90 [Cited within: 1] [JCR: 1.941]
22. P. Bouvier, H. C. Gupta, G. J. Lucazeau, J. Phys. Chem. Solids, 62 (2001), pp. 873–879 [Cited within: 1] [JCR: 1.527]
23. A. M. Limarga, J. Iveland , M. Gentleman, D. M. Lipkin, D. R. Clarke, Acta Mater. , 59 (2011), pp. 1162–1167 [Cited within: 1] [JCR: 3.941]
24. R. A. Miller, R. G. Garlick, J. L. Smialek, Am. Ceram. Soc. Bull. , 62 (1983), pp. 1355–1358 [Cited within: 1] [JCR: 0.522]
25. R. Chaim, D. G. Brand on, A. H. Heuer, Acta Metall. , 34 (1986), pp. 1933–1939 [Cited within: 1] [JCR: 0.612] [CJCR: 0.835]
26. M. N. Rahaman, J. R. Gross, R. E. Dutton, H. Wang, Acta Mater. , 54 (2006), pp. 1615–1621 [Cited within: 1] [JCR: 3.941]
27. M. Hillert, J. Am. Ceram. Soc. , 74 (1991), pp. 2005–2006 [Cited within: 1] [JCR: 2.107]
28. M. Hillert, T. Sakuma, Acta Metall. Mater. , 39 (1991), pp. 1111–1115 [Cited within: 1]
29. H. C. Wang, K. L. Lin, J. Mater. Sci. , 26 (1991), pp. 2501–2506 [Cited within: 1] [JCR: 2.163]
30. J. Chevalier, L. Gremillard, A. V. Virkar, D. R. Clarke, J. Am. Ceram. Soc. , 92 (2009), pp. 1901–1920 [Cited within: 1] [JCR: 2.107]
31. M. Yashima, S. Sasaki, M. Kakihana, Y. Yamaguchi, H. Arashi, M. Yoshimura, Acta Crystallogr. B, 50 (1994), pp. 663–672 [Cited within: 1] [JCR: 2.175]